Macaulay Duration, named after economist Frederick Macaulay, is a measure of a bond's sensitivity to interest rate changes. It calculates the weighted average time it takes to receive the bond's cash flows, factoring in present value. The purpose of Macaulay Duration is to provide investors with a precise measure of risk and return trade-off, essential for effective portfolio management. Understanding this concept impacts investors as it helps gauge the potential volatility of a bond or bond portfolio. Macaulay Duration is particularly significant in contexts of fluctuating interest rates. It is a fundamental concept in fixed-income investing, allowing investors to compare bonds with differing maturity dates, coupons, and face values. Ultimately, it guides strategic investment decisions, contributing to risk management and the potential for higher returns. Macaulay Duration is computed through a mathematical formula that accounts for the discounted value of all future cash flows associated with the bond. This includes the periodic coupon payments as well as the bond's maturity value, all adjusted for the time at which they are received. The entire sum of these discounted cash flows is then divided by the current price of the bond to arrive at the Macaulay Duration. The formula accounts for the time value of money by incorporating the periodic yield in the discounting process. This formula shows that each cash flow is weighted by the time at which it is received, reflecting the time value of money. Understanding the formula requires understanding the relationship between cash flows, time, and discount rates. The discount rate used is typically the yield to maturity of the bond. It accounts for the time value of money, where a dollar received today is worth more than a dollar received in the future. Macaulay Duration serves as a link between bond prices and interest rates, as it measures how sensitive a bond's price is to changes in interest rates. It is a measure of the weighted average time until a bond's cash flows are received. As a rule of thumb, the longer the Macaulay Duration, the higher the interest rate risk for a bond. It is a crucial tool for investors and portfolio managers who need to manage the interest rate risk of their bond investments effectively. The concept of Macaulay Duration is based on the principle that bond prices and interest rates move in opposite directions. When interest rates rise, bond prices fall, and when interest rates fall, bond prices rise. Macaulay Duration quantifies this inverse relationship and helps investors understand how much a bond's price will change with a change in interest rates. Investment professionals frequently use Macaulay Duration to manage interest rate risk and align bond portfolio durations with investment horizons. For example, a pension fund with obligations due in 15 years may choose to invest in bonds with a Macaulay Duration of 15 years to match the fund's liabilities. Portfolio immunization is a strategy used by investors to shield their portfolios from interest rate risk. By balancing the duration of assets and liabilities, investors can protect their portfolios from significant losses when interest rates change. The key to successful portfolio immunization is understanding and applying the Macaulay Duration concept effectively. Asset-Liability Management (ALM) is an essential practice in financial institutions, where the objective is to manage the use of assets and cash flows to meet company obligations. Macaulay Duration plays a pivotal role in ALM, as institutions often seek to match the durations of their assets and liabilities to minimize interest rate risk. While Macaulay Duration is the weighted average time until a bond's cash flows are received, modified duration is a derivative of the Macaulay Duration that measures the percentage change in a bond's price for a 1% change in interest rates. Both are measures of a bond's sensitivity to interest rate changes, but they are used in slightly different contexts. Effective Duration takes into consideration the potential changes in cash flows that result from changes in interest rates. This is particularly relevant for bonds with embedded options, such as callable or puttable bonds. In contrast, Macaulay Duration assumes that cash flows do not change with interest rates, making it less appropriate for bonds with embedded options. While Macaulay Duration is straightforward to calculate and understand, it is not as versatile as modified or effective durations for analyzing bonds with complex features or examining how a bond's price will change with a non-parallel shift in the yield curve. Here’s a sample computation using this formula with a bond that has the following characteristics: The bond has a face value (Maturity Value, M) of $1,000 It pays a 5% annual coupon, which equates to an annual coupon payment (C) of $50 The bond's yield (y) is 6% per annum The bond matures in 2 years, so the duration of the bond in periods (n) is 2 The bond is currently selling for $960 Substitute the values into the Macaulay Duration formula: Macaulay Duration = [∑ (1 + y)^t * t * C + (1 + y)^n * n * M] / Current Bond Price = [(1+0.06)^1 * 1 * 50 + (1+0.06)^2 * 2 * 50 + (1+0.06)^2 * 2 * 1000] / 960 To simplify the calculation, let's calculate each term separately. First term = (1.06)^1 * 1 * 50 = 53 Second term = (1.06)^2 * 2 * 50 = 112.36 Third term = (1.06)^2 * 2 * 1000 = 2247.2 Now, add the three terms and divide by the current bond price: Macaulay Duration = (53 + 112.36 + 2247.2) / 960 = 2.42 years This indicates that, on average, it will take about 2.42 years to receive the cash flows from this bond. For a coupon-paying bond, the calculation method remains largely similar, with one crucial difference: each coupon payment is accounted for as an individual cash flow. This addition underscores the significance of understanding the varying cash inflows associated with a bond's lifespan. Regarding bond portfolios, the computation of Macaulay Duration necessitates an extra step. A weighted average of the Macaulay Durations of the individual bonds is taken, with each bond's weight being determined by its proportion of the portfolio's overall value. Consequently, this approach respects the diverse values and durations of individual bonds within a comprehensive portfolio, providing a more accurate duration measure for the entire portfolio. The Macaulay Duration of a bond is directly related to the bond's price sensitivity to changes in interest rates. A bond with a longer Macaulay Duration will have a greater price change for a given change in interest rates than a bond with a shorter Macaulay Duration. As interest rates increase, the price of a bond decreases, and vice versa. This relationship is more pronounced for bonds with longer Macaulay Durations. Hence, if interest rates are expected to rise, an investor may prefer bonds with shorter Macaulay Durations to limit price volatility. By understanding the concept of Macaulay Duration, investors can better manage the risk and return profile of their bond investments. For example, if investors expect interest rates to decrease, they may want to invest in bonds with longer durations to maximize price appreciation. In a low-interest-rate environment, bonds with longer Macaulay Durations tend to perform better as they have a higher sensitivity to changes in interest rates and can thus provide higher price appreciation. In a high-interest-rate environment, bonds with shorter Macaulay Durations may be more desirable as they are less sensitive to changes in interest rates, protecting investors from significant price drops. Macaulay Duration can change with market conditions, especially for bonds with embedded options. If interest rates change significantly, the issuer or bondholders may choose to exercise their options, changing the bond's cash flows and thus its Macaulay Duration. Macaulay Duration, named after economist Frederick Macaulay, is an indispensable tool for gauging bond sensitivity to interest rate fluctuations. It considers the time value of money and the present value of future cash flows, helping investors manage risk-return trade-offs effectively. This tool plays a pivotal role in asset-liability management, portfolio immunization, and aligning investment horizons with bond durations. Its versatility extends to comparing bonds with varied maturities, coupons, and face values. Although Macaulay Duration is a valuable indicator for simple bonds, modified or effective durations are more suited to complex bond features and non-parallel shifts in the yield curve. Macaulay Duration is a robust, dynamic, and essential concept that helps investors make strategic decisions in various market conditions, enhancing portfolio management and fostering informed investment strategies.Macaulay Duration Overview
Mathematical Explanation of Macaulay Duration
Formula for Calculating Macaulay Duration
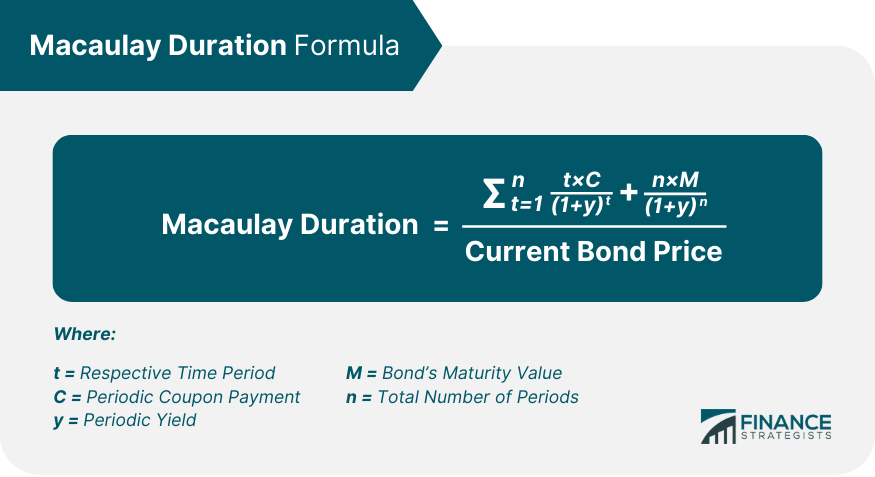
Understanding the Components of the Formula
Conceptual Understanding of Macaulay Duration
Link Between Macaulay Duration and Interest Rates
Macaulay Duration as a Measure of Bond's Interest Rate Risk
Inverse Relationship Between Bond Prices and Interest Rates
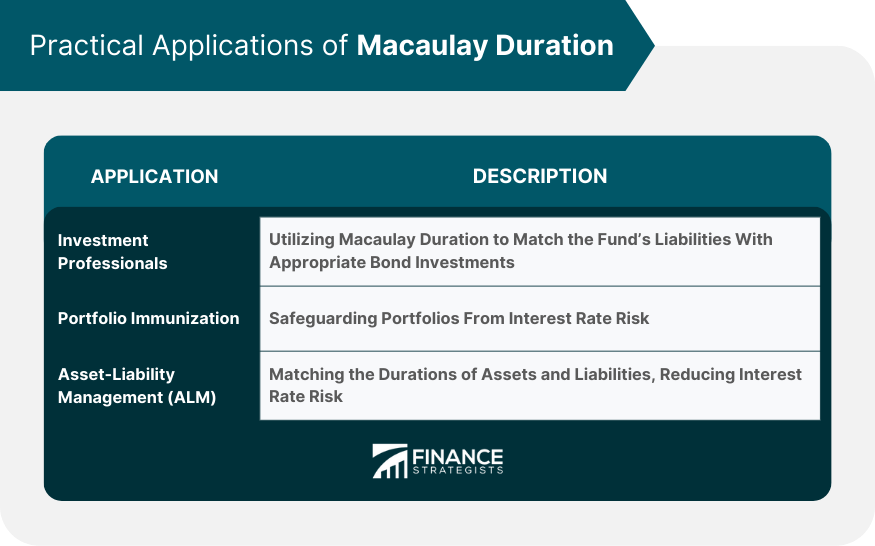
Practical Applications of Macaulay Duration
How Investment Professionals Use Macaulay Duration
Portfolio Immunization and Macaulay Duration
Role of Macaulay Duration in Asset-Liability Management
Comparison of Macaulay Duration With Other Duration Measures
Modified Duration
Effective Duration
Strengths and Limitations of Different Duration Measures
Sample Calculations of Macaulay Duration
Step-By-Step Process for a Simple Bond
Calculating Macaulay Duration for a Coupon-Paying Bond
Macaulay Duration Computation for a Bond Portfolio
Impact of Macaulay Duration on Bond Pricing
Relationship Between Macaulay Duration and Bond Prices
Impact of Changes in Interest Rates on Bonds With Different Macaulay Durations
How Investors Can Use Macaulay Duration to Make Investment Decisions
Macaulay Duration in Current Market Conditions
Low-Interest Rate Environment
High-Interest Rate Environment
Impact of Changing Market Conditions
Final Thoughts
Macaulay Duration FAQs
Macaulay Duration is a measure of a bond's sensitivity to interest rate changes, calculating the weighted average time it takes to receive the bond's cash flows, factoring in present value. It helps investors manage the risk-return trade-off and understand potential volatility in a bond or bond portfolio, especially in fluctuating interest rate conditions.
Macaulay Duration is calculated using a formula that includes the discounted value of all future cash flows from the bond, including periodic coupon payments and the bond's maturity value. The entire sum of these discounted cash flows is divided by the current bond price to give the Macaulay Duration. The formula is: Macaulay Duration = [∑ (1 + y)^t * t * C + (1 + y)^n * n * M] / Current Bond Price.
Macaulay Duration serves as a link between bond prices and interest rates, measuring how sensitive a bond's price is to changes in interest rates. It's based on the principle that bond prices and interest rates move in opposite directions. A longer Macaulay Duration implies a higher interest rate risk for a bond.
Macaulay Duration is used by investment professionals for interest rate risk management, aligning bond portfolio durations with investment horizons, and portfolio immunization. It is also used in Asset-Liability Management (ALM) to match the durations of assets and liabilities, thereby reducing interest rate risk.
Macaulay Duration is the weighted average time until a bond's cash flows are received. Modified Duration, a derivative of Macaulay Duration, measures the percentage change in a bond's price for a 1% change in interest rates. Effective Duration takes into account potential changes in cash flows due to changes in interest rates, which is relevant for bonds with embedded options. Macaulay Duration assumes that cash flows do not change with interest rates.
True Tamplin is a published author, public speaker, CEO of UpDigital, and founder of Finance Strategists.
True is a Certified Educator in Personal Finance (CEPF®), author of The Handy Financial Ratios Guide, a member of the Society for Advancing Business Editing and Writing, contributes to his financial education site, Finance Strategists, and has spoken to various financial communities such as the CFA Institute, as well as university students like his Alma mater, Biola University, where he received a bachelor of science in business and data analytics.
To learn more about True, visit his personal website or view his author profiles on Amazon, Nasdaq and Forbes.











