Net Present Value is a financial metric used to determine the value of an investment by calculating the difference between the present value of cash inflows and the present value of cash outflows over a specified period. NPV is an essential tool for financial decision-making because it helps investors, business owners, and financial managers determine the profitability and viability of potential investments or projects. By comparing NPVs, decision-makers can identify the most attractive investment opportunities and allocate resources accordingly. The time value of money is a fundamental concept in finance, which suggests that a dollar received today is worth more than a dollar received in the future. This concept is the foundation of NPV calculations, as it emphasizes the importance of considering the timing and magnitude of cash flows when evaluating investment opportunities. To calculate NPV, accurate cash flow projections are necessary. These projections estimate the future inflows and outflows associated with a particular investment or project. The accuracy of these projections is crucial, as they directly impact the reliability of the NPV calculation. The discount rate is the rate of return required by an investor for assuming the risk associated with an investment or project. It is used to discount future cash flows back to their present value, ensuring that the time value of money is appropriately considered in NPV calculations. The first step in calculating NPV is identifying the initial investment costs and future cash flows associated with the investment or project. These cash flows should include both inflows (revenues, cost savings, etc.) and outflows (expenses, taxes, etc.). Next, determine the appropriate discount rate, which should reflect the risk associated with the investment or project. This rate can be based on the investor's required rate of return or the weighted average cost of capital (WACC) for the company undertaking the project. Using the discount rate, calculate the present value of each cash flow by dividing the cash flow by (1 + discount rate) raised to the power of the period in which the cash flow occurs. This calculation will provide the present value of each cash flow, adjusted for the time value of money. Finally, subtract the initial investment from the sum of the present values of all cash flows to determine the NPV of the investment or project. A positive NPV indicates that the investment or project is expected to generate a net gain in value, making it an attractive opportunity. The higher the positive NPV, the more profitable the investment or project is likely to be. A negative NPV indicates that the investment or project is expected to result in a net loss in value, making it an unattractive opportunity. In this case, decision-makers should consider alternative investments or projects with higher NPVs. A zero NPV implies that the investment or project will neither generate a net gain nor a net loss in value. In this situation, decision-makers should carefully weigh the risks and potential benefits of the investment or project before making a decision. NPV is widely used in capital budgeting to evaluate the profitability of potential investments in long-term assets, such as machinery, equipment, and real estate. NPV can be used to assess the viability of various projects within a company, comparing their expected profitability and aiding in the decision-making process for project prioritization and resource allocation. Investors use NPV to evaluate potential investment opportunities, such as stocks, bonds, or real estate, to determine which investments are likely to generate the highest returns. NPV is also applied in the valuation of securities, such as bonds, by calculating the present value of their future cash flows and comparing it to the current market price. One of the primary advantages of NPV is its consideration of the time value of money, which ensures that cash flows are appropriately adjusted for their timing and value. NPV takes into account both the magnitude and timing of cash flows, providing a more accurate representation of an investment or project's profitability compared to other methods that may not consider these factors. NPV allows for easy comparison of various investment alternatives or projects, helping decision-makers identify the most attractive opportunities and allocate resources accordingly. The reliability of NPV calculations is highly dependent on the accuracy of cash flow projections. Inaccurate projections can lead to misleading NPV results and suboptimal decision-making. NPV is sensitive to changes in the discount rate, which can significantly impact the results. Small changes in the discount rate can lead to large variations in NPV, making it challenging to determine the optimal investment or project. Comparing NPVs of projects with different lifespans can be problematic, as it may not adequately account for the difference in the duration of benefits generated by each project. IRR is another widely used metric for investment evaluation. It is the discount rate at which the NPV of an investment or project equals zero. Higher IRRs indicate more profitable opportunities. The payback period is the time required for an investment or project to recoup its initial costs. Shorter payback periods are generally more attractive, as they indicate faster recovery of the initial investment. The profitability index is the ratio of the present value of cash inflows to the present value of cash outflows. A profitability index greater than one indicates a profitable investment or project. Net Present Value is a critical tool in financial decision-making, as it enables investors and financial managers to evaluate the profitability and viability of potential investments or projects. By considering the time value of money and the magnitude and timing of cash flows, NPV provides valuable insights for resource allocation and investment prioritization. While NPV offers numerous benefits, it is essential to recognize its limitations, such as its dependence on accurate cash flow projections and sensitivity to discount rate changes. Decision-makers should consider these factors and potentially incorporate alternative evaluation methods, such as IRR, payback period, or profitability index, to ensure well-informed investment and project decisions.What Is Net Present Value (NPV)?
Basics of Net Present Value Calculation
Time Value of Money
Cash Flow Projections
Discount Rate
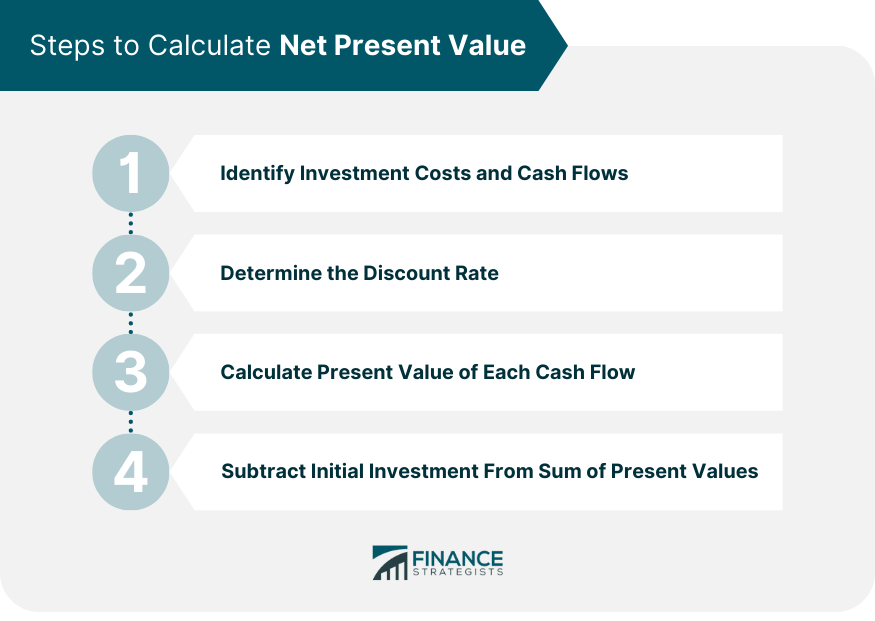
Steps to Calculate Net Present Value
Identify Investment Costs and Cash Flows
Determine the Discount Rate
Calculate Present Value of Each Cash Flow
Subtract Initial Investment From Sum of Present Values
Interpretation of Net Present Value Results
Positive NPV
Negative NPV
Zero NPV
Applications of Net Present Value
Capital Budgeting
Project Evaluation
Investment Appraisal
Valuation of Securities

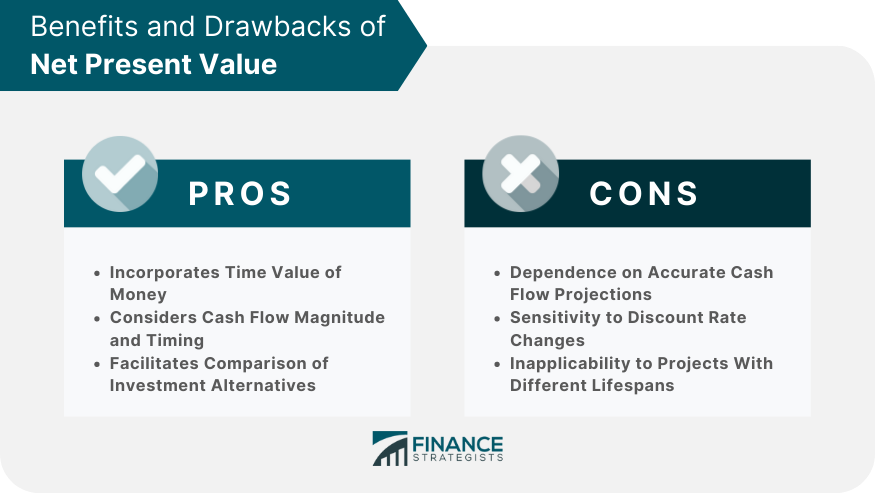
Advantages of Net Present Value
Incorporates Time Value of Money
Considers Cash Flow Magnitude and Timing
Facilitates Comparison of Investment Alternatives
Limitations of Net Present Value
Dependence on Accurate Cash Flow Projections
Sensitivity to Discount Rate Changes
Inapplicability to Projects With Different Lifespans
Alternative Investment Evaluation Methods
Internal Rate of Return (IRR)
Payback Period
Profitability Index
Conclusion
Net Present Value (NPV) FAQs
Net present value is a financial calculation used to determine the present value of future cash flows. It takes into account the time value of money, which means that a dollar today is worth more than a dollar received in the future.
The formula for calculating NPV involves taking the present value of future cash flows and subtracting the initial investment. The present value is calculated by discounting future cash flows using a discount rate that reflects the time value of money.
NPV is an important tool in financial decision-making because it helps to determine whether a project or investment will generate a positive or negative return. If the NPV is positive, it indicates that the investment is expected to generate more cash flows than the initial investment and is therefore a good investment. If the NPV is negative, it indicates that the investment is not expected to generate enough cash flows to cover the initial investment and is therefore a bad investment.
One limitation of NPV is that it relies on accurate cash flow projections, which can be difficult to predict. It also assumes that cash flows will be received at regular intervals, which may not always be the case. Additionally, NPV does not take into account non-financial factors such as risk, which can also impact investment decisions.
The discount rate used in NPV calculations is a critical factor in determining the result. A higher discount rate will result in a lower NPV, while a lower discount rate will result in a higher NPV. This is because a higher discount rate reflects a higher opportunity cost of investing in the project, while a lower discount rate reflects a lower opportunity cost.
True Tamplin is a published author, public speaker, CEO of UpDigital, and founder of Finance Strategists.
True is a Certified Educator in Personal Finance (CEPF®), author of The Handy Financial Ratios Guide, a member of the Society for Advancing Business Editing and Writing, contributes to his financial education site, Finance Strategists, and has spoken to various financial communities such as the CFA Institute, as well as university students like his Alma mater, Biola University, where he received a bachelor of science in business and data analytics.
To learn more about True, visit his personal website or view his author profiles on Amazon, Nasdaq and Forbes.











